欢迎来到奇葩栖息地!欢迎加入Discord服务器:XfrfHCzfbW。请先至特殊:参数设置验证邮箱后再进行编辑。在特殊:参数设置挑选自己想要使用的小工具!不会编辑?请至这里学习Wikitext语法。
物竞小黑板
这是一个巨佬创建的页面,目的是为了帮助大家热爱物理竞赛,欢迎大家一起编辑,也请大家帮助指出其中的错误。
“高考易错题”
谈到高考易错题,大多数人想到的是学生容易做错的题目。但我们这里讨论一些老师容易出错的题目。
例1
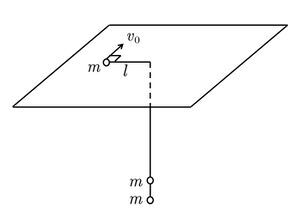
在一个光滑水平面上有一孔,中间穿过一根轻绳,桌面上的一段连接一个质量为[math]\displaystyle{ m }[/math]的球,桌面下的一段连接两个质量为[math]\displaystyle{ m }[/math]的球,桌面上绳长初态为[math]\displaystyle{ l }[/math]。桌面上的球以切向速度[math]\displaystyle{ v_0=\sqrt{gl} }[/math]绕孔做圆周运动。现在释放绳,问桌面上绳最短为多长?
我们设桌面上的绳缩短量为[math]\displaystyle{ x }[/math],则我们需要算出[math]\displaystyle{ x_\rm{max} }[/math]的值。
如果这题被老师一时兴起出到高考试卷上,那么解题过程应该是这样的:
由能量守恒:[math]\displaystyle{ \frac{1}{2}mv_0^2 = \frac{1}{2}mv_1^2 - 2mgx }[/math] ①
由牛顿第二定律:[math]\displaystyle{ \frac{mv_1^2}{l - x} = 2mg }[/math] ②
然而这样做是不对的。这里默认了[math]\displaystyle{ x_\rm{max} }[/math]时沿绳方向的加速度只有向心加速度。事实上,此时沿绳不止有向心加速度,桌面下方的[math]\displaystyle{ 2m }[/math]会再次上升,原因在最后会解释。
那我们怎样解决这个问题呢?我们可以使用角动量。
由[math]\displaystyle{ \vec{F} = m \vec{a} }[/math]:
[math]\displaystyle{ \vec{r} \times \vec{F} \cdot \Delta t = \vec{r} \times m \vec{a} \cdot \Delta t }[/math]
[math]\displaystyle{ \vec{M} \cdot \Delta t = \vec{r} \times m \Delta \vec{v} }[/math]
又[math]\displaystyle{ \Delta (\vec{r} \times m \vec{v}) }[/math]
[math]\displaystyle{ = \Delta \vec{r} \times m \vec{v} + \vec{r} \times \Delta (m \vec{v}) }[/math]
[math]\displaystyle{ = \vec{v} \cdot \Delta t \times m \vec{v} + \vec{r} \times m \Delta \vec{v} }[/math]
[math]\displaystyle{ = 0 + \vec{r} \times m \Delta \vec{v} }[/math]
所以[math]\displaystyle{ \vec{M} \cdot \Delta t = \Delta (\vec{r} \times m \vec{v}) }[/math]
记作[math]\displaystyle{ \vec{H} = \Delta \vec{L} }[/math]
其中,[math]\displaystyle{ \vec{H} }[/math]是角冲量,[math]\displaystyle{ \vec{L} }[/math]是角动量。
在此题中,使用角动量是好的。因为桌面上的小球受到绳的力是有心力,即:若选孔为支点,则运动过程中绳上拉力力臂始终为0,力矩为0,角动量守恒。
由此,得方程:[math]\displaystyle{ m v_0 l = m v_1 (l-x) }[/math] ③
联立①③,得[math]\displaystyle{ x_\rm{max}=\frac{7-\sqrt{17}}{8} }[/math][math]\displaystyle{ l }[/math]
由这个结果不难发现,[math]\displaystyle{ x_\rm{max} }[/math]时,向心加速度为[math]\displaystyle{ \frac{-13+5\sqrt{17}}{2}g \gt 2g }[/math],说明我们之前的想法是正确的。
这个问题就轻松而愉快地解决了!
| 数学 |
| ||||
|---|---|---|---|---|---|
| 物理 |
| ||||
| 化学 |
| ||||
| 信息学 |
| ||||
| 生物学 |
| ||||
| 其他 | |||||
