欢迎来到奇葩栖息地！欢迎加入Discord服务器：XfrfHCzfbW。请先至特殊:参数设置验证邮箱后再进行编辑。在特殊:参数设置挑选自己想要使用的小工具！不会编辑？请至这里学习Wikitext语法。

代微积拾级：修订间差异

来自奇葩栖息地
交互式浏览历史
添加的内容 删除的内容
可视化wikitext
第1,405行： 第1,405行：
English=
English=
原文如下：
原文如下：
{{from|A<span style{{=}}"font-size: 70%;>RTICLE</span> (157.) I<span style{{=}}"font-size: 70%;>N</span> the Differential Calculus, as in Analytical Geometry, there are two classes of quantities to be considered, viz., ''variables'' and ''constants''.<br><br>Variable quantities are generally represented by the last letters of the alphabet, ''x'', ''y'', ''z'', etc., and any values may be assigned to them which will satisfy the equations into which they enter.<br><br>Constant quantities are generally represented by the firstletters of the alphabet, ''a'', ''b'', ''c'', etc., and these always retain the same values throughout the same investigation.<br><br>Thus, in the equation of a straight line, <br><div style="text-align: center;"><math>y=ax+b</math>,</div>the quantities a and b have but one value for the same line, while ''x'' and ''y'' vary in value for every point of the line.<br><br>(158.) '''One variable is said to be a ''function'' of another variable, when the first is equal to a certain algebraic expression containing the second.''' Thus, in the equation of a straight line <br><div style="text-align: center;"><math>y=ax+b</math>,</div>''y'' is a function of ''x''. <br><br>So, also, in the equation of a circle, <br><div style="text-align: center;"><math>y=\sqrt{R^2-x^2}</math>;</div>and in the equation of the ellipse, <br><div style="text-align: center;"><math>y=\frac{B}{A}\sqrt{2Ax-x^2}</math>.</div>(159.) When we wish merely to denote that ''y'' is ''dependent'' upon ''x'' for its value, without giving the particular expression which shows the value of ''x'', we employ the notation<br><div style="text-align: center;"><math>y=F(x)</math>, or <math>y=f(x)</math>,<br><span style{{=}}"text-align: left; float: left;">or</span><math>x=F(y)</math>, or <math>x=f(y)</math>,</div>which expressions are read, ''y'' is a function of ''x'', or ''x'' is a function of ''y''.}}
{{from|1=A<span style{{=}}"font-size: 70%;>RTICLE</span> (157.) I<span style{{=}}"font-size: 70%;>N</span> the Differential Calculus, as in Analytical Geometry, there are two classes of quantities to be considered, viz., ''variables'' and ''constants''.<br><br>Variable quantities are generally represented by the last letters of the alphabet, ''x'', ''y'', ''z'', etc., and any values may be assigned to them which will satisfy the equations into which they enter.<br><br>Constant quantities are generally represented by the firstletters of the alphabet, ''a'', ''b'', ''c'', etc., and these always retain the same values throughout the same investigation.<br><br>Thus, in the equation of a straight line, <br><div style="text-align: center;"><math>y=ax+b</math>,</div>the quantities a and b have but one value for the same line, while ''x'' and ''y'' vary in value for every point of the line.<br><br>(158.) '''One variable is said to be a ''function'' of another variable, when the first is equal to a certain algebraic expression containing the second.''' Thus, in the equation of a straight line <br><div style="text-align: center;"><math>y=ax+b</math>,</div>''y'' is a function of ''x''. <br><br>So, also, in the equation of a circle, <br><div style="text-align: center;"><math>y=\sqrt{R^2-x^2}</math>;</div>and in the equation of the ellipse, <br><div style="text-align: center;"><math>y=\frac{B}{A}\sqrt{2Ax-x^2}</math>.</div>(159.) When we wish merely to denote that ''y'' is ''dependent'' upon ''x'' for its value, without giving the particular expression which shows the value of ''x'', we employ the notation<br><div style="text-align: center;"><math>y=F(x)</math>, or <math>y=f(x)</math>,<br><span style{{=}}"text-align: left; float: left;">or</span><math>x=F(y)</math>, or <math>x=f(y)</math>,</div>which expressions are read, ''y'' is a function of ''x'', or ''x'' is a function of ''y''.|2=''Elements of Analytical Geometry and of The Differential and Integral Calculus'', Differential Calculus, Section I, Definitions and First Principles}}


<div style="text-align: center;">[[File:Elements of Analytical Geometry and of The Differential and Integral Calculus.pdf|page=124|300px]][[File:Elements of Analytical Geometry and of The Differential and Integral Calculus.pdf|page=125|300px]]</div>
<div style="text-align: center;">[[File:Elements of Analytical Geometry and of The Differential and Integral Calculus.pdf|page=124|300px]][[File:Elements of Analytical Geometry and of The Differential and Integral Calculus.pdf|page=125|300px]]</div>

2024年3月10日 (日) 07:19的版本

此页面使用了SimpleMathJax扩展渲染的TEX公式。

这些公式被包含在<math>（包括$或\(\)及不换行的\[\]）或<chem>标签中，请稍等片刻等待前端渲染。
关于在wiki上如何使用TEX，请参见帮助:数学公式。

代微积拾级
作者
伟烈亚力口译
李善兰笔述
伊莱亚斯·罗密士原著
出版时间
1859，清咸丰九年（己未）
1851（原著）
出版社
墨海书馆
“

米利堅羅密士譔，英國偉烈亞力口譯，海寧李善蘭筆述。

”
——《代微積拾級》

《代微积拾（shè）级》，由英国汉学家、来华传教士伟烈亚力（Alexander Wylie，1815年4月6日－1887年2月10日）口译，清代数学家李善兰（1811年1月22日－1882年12月9日）笔述；1859年由上海墨海书馆（The London Missionary Society Press）出版。原著为美国数学家伊莱亚斯·罗密士（Elias Loomis，1811年8月7日－1889年8月15日）于1851年出版的《解析几何和微积分初步》（Elements of Analytical Geometry and of The Differential and Integral Calculus）。

据考证，李善兰翻译所用的底本，应为1852年再版的版本，而非1851年的初版（内容差别较大）。原著在1874年改版，在此之前有不下20个版次（包括1856年的第6版、1859年的第10版、1864年的第17版、1868年的第19版等），畅销程度可见一斑。

1872年（明治5年），这本书被福田半译至日文，但书中并未使用李善兰创立的对应符号系统（参见#符号），而是使用原著的符号。

需要注意的是，这本书中的定义以今天的眼光来看并不严谨。一方面，当时的微积分学基础并不牢固，(ε, δ)-极限定义还未出现，书中仍然只使用未严格定义的无穷小量来定义概念；另一方面，这本书本身就是入门教材，不应过于晦涩难懂。

  • 代（解析几何）

    代（解析几何）

  • 微（微分）

    微（微分）

  • 积（积分）

    积（积分）

作者

李善蘭，字壬叔，海寧人。諸生。從陳奐受經，於算術好之獨深。十歲即通九章，後得測圓海鏡、句股割圜記，學益進。疑割圜法非自然，精思得其理。嘗謂道有一貫，藝亦然。測圓海鏡每題皆有法有草，法者，本題之法也；草者，用立天元一曲折以求本題之法，乃造法之法，法之源也。算術大至躔離交食，細至米鹽瑣碎，其法至繁，以立天元一演之，莫不能得其法。故立天元一者，算學中之一貫也。並時明算如錢塘戴煦，南匯張文虎，烏程徐有壬、汪曰楨，歸安張福僖，皆相友善。咸豐初，客上海，識英吉利偉烈亞力、艾約瑟、韋廉臣三人，偉烈亞力精天算，通華言。善蘭以歐幾里幾何原本十三卷、續二卷，明時譯得六卷，因與偉烈亞力同譯後九卷，西士精通幾何者尟，其第十卷尤玄奧，未易解，譌奪甚多，善蘭筆受時，輒以意匡補。譯成，偉烈亞力歎曰：「西士他日欲得善本，當求諸中國也！」

偉烈亞力又言美國天算名家羅密士嘗取代數、微分、積分合為一書，分款設題，較若列眉，復與善蘭同譯之，名曰代微積拾級十八卷。代數變天元、四元，別為新法，微分、積分二術，又藉徑於代數，實中土未有之奇秘。善蘭隨體剖析自然，得力於海鏡為多。

粵匪陷吳、越，依曾國籓軍中。同治七年，用巡撫郭嵩燾薦，徵入同文館，充算學總教習、總理衙門章京，授戶部郎中、三品卿銜。課同文館生以海鏡，而以代數演之，合中、西為一法，成就甚眾。光緒十年，卒於官，年垂七十。

善蘭聰彊絕人，其於算，能執理之至簡，馭數至繁，故衍之無不可通之數，抉之即無不可窮之理。所著則古昔齋算學，詳藝文志。世謂梅文鼎悟借根之出天元，善蘭能變四元而為代數，蓋梅氏後一人云。

[取自《清史稿·列傳二百九十四·疇人二》]

影印本

符号

字母

符号表，注意希腊字母Ρ与Μ对应颠倒

在书中，小写拉丁字母以天干（10个）、地支（12个）和物（w）、天（x）、地（y）、人（z）对应；小写希腊字母以二十八宿的前24个对应。大写字母将小写字母对应的汉字左侧加上“口”来表示，即使加上口字旁的字已存在，也按照小写的读音来读（例如小写的v对应“亥”，那么大写的V对应的“咳”也读作hài）。另外还有一些专用的符号单独对应。

符号对照表
A 呷（jiǎ） a 甲 Α 唃（jiǎo） α 角（jiǎo） F 㖤（hán）
B 𠮙（yǐ） b 乙 Β 吭（kàng） β 亢（kàng） f 函
C 𠰳（bǐng） c 丙 Γ 呧（dǐ） γ 氐（dǐ） ϕ 𭡝（hán）
D 叮 d 丁 Δ δ 房 ψ 涵
E 𱒐（wù） e 戊 Ε 吣（xīn） M 根
F 𠯇（jǐ） f 己 Ζ 𠳿（wěi） ζ 尾 π 周[表注 1]
G 𱓒（gēng） g 庚 Η 𱕍（jī） η 箕（jī） ε 訥（nè）（讷）[表注 2]
H 㖕（xīn） h 辛 Θ 呌（dǒu） θ 斗（dǒu） d 彳（wēi）[表注 3]
I 𠰃（rén） i 壬 Ι 吽（níu） ι 牛 ∫ 禾（jī）[表注 4]
J 𱓩（guǐ） j 癸 Κ 𠯆（nǚ） κ 女
K 吇（zǐ） k 子 Λ 嘘（xū） λ 虛
L 吜（choǔ） l 丑 Μ 𠱓（wēi）[表注 5] μ 危
M 𠻤（yín） m 寅 Ν 㗌（shì） ν 室
N 𠰭（mǎo） n 卯 Ξ ξ 壁
O 㖘（chén） o 辰 Ο 喹（kuí） ο 奎
P 𱒄（sì） p 巳 Π 嘍（lóu）（喽）
Q 吘（wǔ） q 午 Ρ 喟（wèi）[表注 6] ρ 胃
R 味 r 未 Σ 𭈾（mǎo） σ 昴（mǎo）
S 呻 s 申 Τ 嗶（bì）（哔） τ 畢（毕）
T 唒（yǒu） t 酉 Υ 嘴（zī） υ 觜（zī）
U 㖅（xù） u 戌 Φ 嘇（shēn）（𰇼）
V 咳（hài） v 亥 Χ 𠯤（jǐn） χ 井
W 𭈘（wù） w 物 Ψ 𠺌（guǐ）
X 𱒆（tiān） x 天 Ω ω 柳
Y 哋（dì） y 地
Z 㕥（rén） z 人

运算符及其他引进符号

LaTeX HTML 说明
[math]\displaystyle{ \bot }[/math][表注 7] ⊥[表注 8] 正也，加也[表注 9]
[math]\displaystyle{ \top }[/math][表注 10] ⊤[表注 11] 負也，減也[表注 12]
[math]\displaystyle{ \times }[/math][表注 13] ×[表注 14] 相乘也
[math]\displaystyle{ \div }[/math][表注 15] ÷[表注 16] 約也，或作[math]\displaystyle{ - }[/math]
[math]\displaystyle{ :::: }[/math] :::: 四率比例也
[math]\displaystyle{ () }[/math] () 括諸數為一數也，名曰括弧
[math]\displaystyle{ \sqrt{} }[/math][表注 17] √ 開方根也
[math]\displaystyle{ = }[/math] = 等於[表注 18]
[math]\displaystyle{ \lt }[/math] < 右大於左
[math]\displaystyle{ \gt }[/math] > 左大於右
[math]\displaystyle{ 0 }[/math] 0 無也
[math]\displaystyle{ \infty }[/math][表注 19] ∞ 無窮也

注释

  1. ↑ 指圆周率。
  2. ↑ 指自然常数，疑似应为e。
  3. ↑ 音同“微”，指微分号。
  4. ↑ 音同“积”，指积分号。
  5. ↑ 书中表格与Ρ颠倒。
  6. ↑ 书中表格与Μ颠倒。
  7. ↑ \bot
  8. ↑ U+22A5，&bottom;&bot;&perp;&UpTee;&#8869;
  9. ↑ 此处并非垂直符号[math]\displaystyle{ \perp }[/math]（\perp）或⟂（U+27C2，&#10178;）。不使用加号+（U+002B，&#plus;&#43;）是因为易与汉字数字“十”混淆。此为“上”的古字（丄，U+4E04，&#19972;）。
  10. ↑ \top
  11. ↑ U+22A4，&top;&DownTee;&#8868;
  12. ↑ 不使用减号−（U+2212，&minus;&#8722;）或连字暨减号-（U+002D，&#45;）是因为易与汉字数字“一”混淆。此为“下”的古字（丅，U+4E05，&#19973; ）。
  13. ↑ \times
  14. ↑ U+00D7，&times;&#215;
  15. ↑ \div
  16. ↑ U+00F7，&divide;&#247;
  17. ↑ \sqrt{}
  18. ↑ 与原符号不同，书中为防止与汉字数字“二”混淆而被拉长。
  19. ↑ \infty

术语

书中新创了大量术语，其中有很多沿用至今。

函数

在卷十的开头，可以看到那句经典名句：

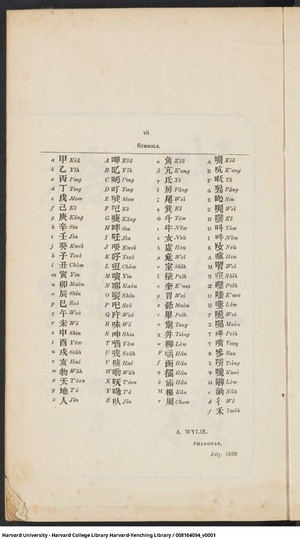
微分之數有二，一曰常數，一曰變數；變數以天地人物等字代之，常數以甲乙子丑等字代之。

凡式中常數之同數俱不變。如直線之式爲[math]\displaystyle{ 地\xlongequal{\qquad}甲天\bot乙 }[/math]，則線之甲與乙，俱僅有一同數任在何點永不變，而天與地之同數則每點皆變也。

凡此變數中函彼變數，則此爲彼之函數。

如直線之式爲[math]\displaystyle{ 地\xlongequal{\qquad}甲天\bot乙 }[/math]，則地爲天之函數；又平圜之式爲[math]\displaystyle{ 地\xlongequal{\qquad}\sqrt{味^二\top甲^二} }[/math]，味爲半徑，天爲正弦，地爲餘弦；橢圓之式爲[math]\displaystyle{ 地\xlongequal{\qquad} }[/math]呷/𠮙[math]\displaystyle{ \sqrt{二呷天\top天^二} }[/math]，皆地爲天之函數也。

設不明顯天之函數，但指地爲天之因變數，則如下式[math]\displaystyle{ 天\xlongequal{\qquad}函(地) \ 地\xlongequal{\qquad}函(天) }[/math]，此天爲地之函數，亦地爲天之函數。

[取自《代微積拾級·卷十 微分一·例》]

原文如下：

ARTICLE (157.) IN the Differential Calculus, as in Analytical Geometry, there are two classes of quantities to be considered, viz., variables and constants.

Variable quantities are generally represented by the last letters of the alphabet, x, y, z, etc., and any values may be assigned to them which will satisfy the equations into which they enter.

Constant quantities are generally represented by the firstletters of the alphabet, a, b, c, etc., and these always retain the same values throughout the same investigation.

Thus, in the equation of a straight line,
[math]\displaystyle{ y=ax+b }[/math],
the quantities a and b have but one value for the same line, while x and y vary in value for every point of the line.

(158.) One variable is said to be a function of another variable, when the first is equal to a certain algebraic expression containing the second. Thus, in the equation of a straight line
[math]\displaystyle{ y=ax+b }[/math],
y is a function of x.

So, also, in the equation of a circle,
[math]\displaystyle{ y=\sqrt{R^2-x^2} }[/math];
and in the equation of the ellipse,
[math]\displaystyle{ y=\frac{B}{A}\sqrt{2Ax-x^2} }[/math].
(159.) When we wish merely to denote that y is dependent upon x for its value, without giving the particular expression which shows the value of x, we employ the notation
[math]\displaystyle{ y=F(x) }[/math], or [math]\displaystyle{ y=f(x) }[/math],
or[math]\displaystyle{ x=F(y) }[/math], or [math]\displaystyle{ x=f(y) }[/math],
which expressions are read, y is a function of x, or x is a function of y.

[取自Elements of Analytical Geometry and of The Differential and Integral Calculus, Differential Calculus, Section I, Definitions and First Principles]

“凡此变数中函彼变数，则此为彼之函数”（One variable is said to be a function of another variable, when the first is equal to a certain algebraic expression containing the second.）这句话，往往被讹传为出现在李善兰另一译作《代数学》中，其实并非如此，出处就是《代微积拾级》。

微分

同样是卷十，可以看到微分的定义：

函數與變數之變比例俱謂之微分，用彳號記之。如 [math]\displaystyle{ 戌\xlongequal{\qquad}天^三 }[/math]，則得比例 𢓍 : [math]\displaystyle{ :: 一 : 三天^二 }[/math]。𢓍，爲天與戌之微分。后皆仿此，用表天與戌之變比例。以一四兩率相乘，二三兩率相乘，則得[math]\displaystyle{ \xlongequal{\qquad}三天^二 }[/math]𢓍。此顯函數戌之變比例，等于 三天二 乘變數天之變比例。以𢓍約之，得𢓍/[math]\displaystyle{ \xlongequal{\qquad}三天^二 }[/math]。此顯變數之變比例，約函數之變比例，等于函數之微係數也。如戌爲天之函數，三天 爲戌之微係數，此舉立方以㮣其餘。

[取自《代微積拾級·卷十 微分一·論函數微分·第二款》]

原文如下：

Elements of Analytical Geometry and of The Differential and Integral Calculus, Differential Calculus, Section I, Differentiation of Algebraic Functions, Proposition II － Theorem

[取自{{{2}}}]
注意，时至今日，微分号为了与量区别而记作[math]\displaystyle{ \mathrm{d} }[/math]，书中的微分号仍然是斜体[math]\displaystyle{ d }[/math]。

书中所称“Differential Coefficient”，译作“微系数”，今天称作导数（Derivative）。无论是中英文，这种古老的说法都已很罕见。

积分

在卷十七，定义了积分：

積分爲微分之還原，其法之要，在識別微分所由生之函數。如已得 天二 之微分爲 二天𢓍，則有 二天𢓍，即知所由生之函數爲 天二，而 天二 即爲積分。

已得微分所由生之函數爲積分。而積分或有常數附之，或無常數附之，既不能定，故式中恒附以常數，命爲𠰳。𠰳或有同數，或爲〇，須攷題乃知。

來本之視微分，若函數諸小較之一，諸小較并之，即成函數。故微分之左係一禾字，指欲取諸微分之積分也。如下式 禾二天𢓍[math]\displaystyle{ \xlongequal{\qquad} 天^二 \bot }[/math]𠰳。來氏說今西國天算家大率不用，而惟用此禾字，取其一覽了然也。

[取自《代微積拾級·卷十七 積分一·總論》]

原文如下：

Elements of Analytical Geometry and of The Differential and Integral Calculus, Integral Calculus, Section I, Integration of Monomial Differentials

[取自{{{2}}}]

实际上，书中的积分号仅仅写作∫，没有写得像今天的[math]\displaystyle{ \int }[/math]那么长。

学习
数学
认真学习
谈笑风生
物理
认真学习
谈笑风生
化学
认真学习
谈笑风生
信息学
认真学习
谈笑风生
生物学
认真学习
谈笑风生
其他
取自“https://mh.wdf.ink/w/index.php?title=代微积拾级&oldid=3256”