欢迎来到奇葩栖息地!欢迎加入Discord服务器:XfrfHCzfbW。请先至特殊:参数设置验证邮箱后再进行编辑。在特殊:参数设置挑选自己想要使用的小工具!不会编辑?请至这里学习Wikitext语法。
高观点下的高中物理:修订间差异
小 (→导言) |
小 (→毕奥萨伐尔定律:// Edit via InPageEdit) |
||
| 第448行: | 第448行: | ||
对于一个闭合回路的电流圈,在空间中一点处的磁感应强度可以写为: |
对于一个闭合回路的电流圈,在空间中一点处的磁感应强度可以写为: |
||
<math>\vec{B} = \oint_L\frac{\mu_0}{4\pi}\frac{ |
<math>\vec{B} = \oint_L\frac{\mu_0}{4\pi}\frac{Id\vec{l}}{r^2}\times\hat{r}</math> |
||
==== 安培环路定理 ==== |
==== 安培环路定理 ==== |
||
2022年3月23日 (三) 03:38的版本
请帮助我们扩充或改进这篇文章。
这些公式被包含在<math>(包括$或\(\)及不换行的\[\])或<chem>标签中,请稍等片刻等待前端渲染。
关于在wiki上如何使用TEX,请参见帮助:数学公式。
高中物理对于一个问题过分地简单化导致学生们对于物理问题与概念的错误理解,其主要原因是数学不够用。
因此,本页会使用大量高等数学的方法来帮助理解物理的本质。
本页中若不注明,则引用部分中,物理部分大部分来自大学《新概念普通物理》,数学部分大部分来自同济七版的《高等数学》。
力学
运动学
自由度
自由度是运动的基本概念之一,它描述了一个物体在空间中的运动的复杂程度。
定义
一个自由度就是一个单独变量。
比如,直线运动时,可以建立一个类似于数轴的东西描述这个物体在直线上的位置。
所谓独立,就是指变量互相之间没有约束。 例如,圆周运动,或者说二次曲线运动,在平面直角坐标系中,有方程[math]\displaystyle{ f\left(x,y\right)=Ax^2+By^2+Cxy+Dx+Ey+F=0 }[/math]
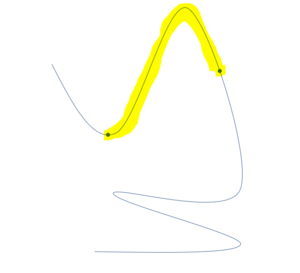
看起来是两个变量,但是这两个变量之间存在约束,因为如果知道其中一个,另一个也能够算出来,所以并不独立。如果以曲线上一点为原点,以质点从原点出发走到该点经过的路程计量,就可以直接描述其位置。
刚体的自由度
一般来说,刚体需要六个自由度才能描述,分别是三个平动自由度,以及三个转动自由度。
取刚体其中一个点的位置为[math]\displaystyle{ \left(x,y,z\right) }[/math],其它点与这个点的相对位置不变(刚体的定义),所以只要取其中一个点,与该点相连,得到一条直线,由于这条直线经过的一个点已经确认,只需要再通过转动自由度确定其方向即可,设这条直线与三个坐标轴的夹角分别为[math]\displaystyle{ \left(\alpha,\beta,\gamma\right) }[/math],其中三个角度必须满足[math]\displaystyle{ \cos^2\alpha+\cos^2\beta+\cos^2\gamma = 1 }[/math]。
运动的描述
位移
位移及运动始末状态的相对位置差,[math]\displaystyle{ \vec{x} = (x_1 - x_2, y_1 - y_2, z_1 - z_2) }[/math].
瞬时速度
速度,相对位置差在时间上的变化率,位移对时间的导数[math]\displaystyle{ \vec{v} = \lim_{\Delta t\to 0}{\frac{\Delta\vec{x}}{\Delta t}}=\frac{d\vec{x}}{dt}=\dot{x} }[/math].
瞬时加速度
加速度,速度在时间上的变化率,速度对时间的导数[math]\displaystyle{ \vec{a} = \lim_{\Delta t\to 0}{\frac{\Delta\vec{v}}{\Delta t}}=\frac{d\vec{v}}{dt}=\ddot{x}=\dot{v} }[/math].
微元法与微积分描述运动
微元法
微元法是将微积分初等化的方法,也是微积分的鼻祖。
微积分太暴力,有时候,微元法更加简便快速。 例如下面这一题两半径相同的圆环,右边的原环以速度[math]\displaystyle{ v }[/math]向左运动,假设两环圆心距离[math]\displaystyle{ d\lt 2R }[/math],两环上方交点为[math]\displaystyle{ A }[/math],求[math]\displaystyle{ v_A }[/math].
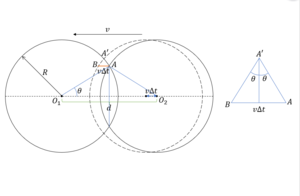
可以假设右环走了[math]\displaystyle{ v\Delta t }[/math],此时[math]\displaystyle{ A }[/math]点移至[math]\displaystyle{ A' }[/math]点,作[math]\displaystyle{ AB // O_1 O_2 }[/math],显然有[math]\displaystyle{ AB = v\Delta t,A'B=A'A = \frac{\frac{1}{2}AB}{\sin\theta}=\frac{v\Delta t}{2\sin\theta} }[/math].
而[math]\displaystyle{ \sin\theta = \frac{\sqrt{R^2-\left(d/2\right)^2}}{R} }[/math]
所以[math]\displaystyle{ v_A\Delta t = A'A = \frac{v\Delta tR}{2\sqrt{R^2-\left(d/2\right)^2}} }[/math]
得到[math]\displaystyle{ v_A = \frac{vR}{\sqrt{4R^2-d^2}} }[/math]
微积分描述运动
微积分就更加直截了当,少了很多论证。 仍用上面的例子,对于微积分,可以直接建立坐标系,暴力求解。
设[math]\displaystyle{ A\left(x(t),y(t)\right) }[/math]
易知[math]\displaystyle{ x(t) = \frac{1}{2}O_1O_2 = \frac{1}{2}\left(2R-vt\right),y(t)=\sqrt{R^2-x^2}=\frac{1}{2}\sqrt{4R^2-\left(2R-vt\right)^2} }[/math]
则[math]\displaystyle{ v_x = \dot{x}(t) = -\frac{v}{2},v_y = \dot{y}(t)=\frac{2vR - v^2t}{2\sqrt{4R^2-\left(2R-vt\right)^2}} }[/math]

当[math]\displaystyle{ O_1O_2 = d }[/math]时,[math]\displaystyle{ t = \frac{2R-d}{v} }[/math],
所以[math]\displaystyle{ v_y=\frac{vd}{2\sqrt{4R^2-d^2}} }[/math]
则[math]\displaystyle{ v_A=\sqrt{v_x^2+v_y^2}=\frac{vR}{\sqrt{4R^2-d^2}} }[/math]
微积分的更广泛应用
导数 微分
因为导数与微分是对一个物理量相对于另一个物理量的变化率的描述,所以物理中应用广泛。
任何物理量之间只要它们的比值的有一定意义,就都可以通过导数微分进行一系列研究。
微分与导数几乎没有什么区别,在数学形式上,微分把导数的分母乘在了等式的另一边:
导数:[math]\displaystyle{ y'(x)=\frac{dy}{dx} }[/math]
微分:[math]\displaystyle{ d\left[y(x)\right]=y'(x)dx }[/math]
一般来说,都是求导更方便直接计算,微分更适合小量分析。
导数:[math]\displaystyle{ \frac{dy}{dx} = y'(x) = \lim_{\Delta x\to 0}{\frac{y(x+\Delta x)-y(x)}{\Delta x}} }[/math]
导数有四则运算、复合函数求导、隐函数求导等等,其中的运算过程越复杂,就越是要用微分进行小量分析,然后简化运算。
位移 速度 加速度
位移函数([math]\displaystyle{ x(t) }[/math]),速度([math]\displaystyle{ v(t)=\dot{x}(t) }[/math])就是其对时间的一阶导数,加速度[math]\displaystyle{ a(t)=\dot{v}(t)=\ddot{x}(t) }[/math]就是对时间的二阶导数,或是速度对时间的一阶导数。
曲率 曲率半径
曲线弧长公式
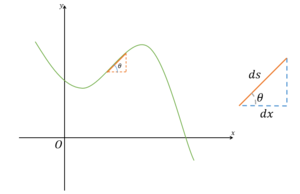
对于一段曲线[math]\displaystyle{ f(x) }[/math],假设其曲线长公式为一关于[math]\displaystyle{ x }[/math]的函数[math]\displaystyle{ s(x) }[/math],
截取其中一小段弧[math]\displaystyle{ ds }[/math]可以认为它是一个小线段,
那么显然就有[math]\displaystyle{ (ds)^2=(dx)^2+(dy)^2 }[/math]
[math]\displaystyle{ =dx^2\left[1+\left(\frac{dy}{dx}\right)^2\right] }[/math]
[math]\displaystyle{ =(dx)^2(1+\tan^2\theta)=(dx)^2(1+y'^2) }[/math]
所以[math]\displaystyle{ ds = \sqrt{1+y'^2}dx }[/math]
于是[math]\displaystyle{ s(x) = \int_{x_1}^{x_2}{\sqrt{1+y'^2}dx} }[/math]
曲率 曲率半径
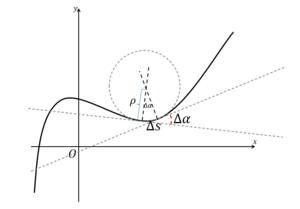
对于曲线C,其弧长随前线偏转角的变化率称为曲率半径[math]\displaystyle{ \rho=\frac{1}{K}=\left|\frac{\Delta s}{\Delta\alpha}\right| }[/math]
易知[math]\displaystyle{ d\left(\tan\alpha\right)=\frac{d\tan\alpha}{d\alpha}d\alpha }[/math]
[math]\displaystyle{ ds=\sqrt{1+y'^2}dx }[/math]
而[math]\displaystyle{ \frac{d\tan\alpha}{d\alpha}=\sec^2\alpha=1+\tan^2\alpha=1+y'^2 }[/math]
所以[math]\displaystyle{ d\alpha=\left(1+y'^2\right)dx }[/math]
所以[math]\displaystyle{ \rho=\left|\frac{\Delta s}{\Delta\alpha}\right|=\frac{\left(1+y'^2\right)^{\frac{3}{2}}}{\left|y''\right|} }[/math]
所以曲率[math]\displaystyle{ K=\frac{\left|y''\right|}{\left(1+y'^2\right)^{\frac{3}{2}}} }[/math]
当然对于参数方程[math]\displaystyle{ x=\varphi(t),y=\psi(t) }[/math]
我们有[math]\displaystyle{ K=\frac{\left|\varphi'\psi''-\varphi''\psi'\right|}{\left(\varphi'^2+\psi'^2\right)^{\frac{3}{2}}} }[/math]
[math]\displaystyle{ \rho=\frac{\left(\varphi'^2+\psi'^2\right)^{\frac{3}{2}}}{\left|\varphi'\psi''-\varphi''\psi'\right|} }[/math]
有了曲率半径,就可以求出一个质点的向心加速度[math]\displaystyle{ a_n=\frac{v_{\tau}^2}{\rho} }[/math]
积分
积分分为不定积分与定积分。
不定积分
不定积分是导数和微分的逆运算,所以可以写成[math]\displaystyle{ \int{F'(x)dx} = F(x) + C }[/math]
由定义可知,不定积分的运算方法就是找出什么函数的导函数等于被积函数。
当然有些时候,并不是那么容易看出来积分函数长什么样子,所以就诞生了一些方法。
一、换元积分法:
第一类换元法:设[math]\displaystyle{ u = \varphi(x) }[/math]
则[math]\displaystyle{ F(x) = \int f\left[\varphi(x)\right]\cdot\varphi'(x)\ dx = \int f(u)\ du }[/math]
第二类换元法:设[math]\displaystyle{ x = \varphi(u) }[/math]
则[math]\displaystyle{ F(x) = \int f(x)\ dx = \int f\left[\varphi(u)\right]\cdot\varphi'(u)\ du }[/math]
二、分部积分法
由求导法则[math]\displaystyle{ (uv)' = u'v + uv' }[/math]可以得到[math]\displaystyle{ uv' = (uv)' - u'v }[/math]
积分一下,就可以得到[math]\displaystyle{ \int uv'\ dx = uv - \int u'v\ dx }[/math]
定积分
定积分:[math]\displaystyle{ \int_a^b f(x)\ dx = F(b) - F(a) }[/math]
位移 速度 加速度
曲线的路径长
对于曲线[math]\displaystyle{ C:y=f(x) }[/math]
有:[math]\displaystyle{ s(x) = \int_{x_1}^{x_2}{\sqrt{1+y'^2}dx} }[/math]
对于参数方程[math]\displaystyle{ x = \varphi(t), y = \psi(t) }[/math]
有:[math]\displaystyle{ s(t) = \int_{t_1}^{t_2}{\sqrt{\varphi'^2(t) + \psi'^2(t)}dt} }[/math]
使用相图简化问题
运动的关联
坐标系
平面直角坐标系
极坐标系
静力学
惯性系
牛顿第一第三定律
平衡的条件
三种平衡及其判定方法
动力学
牛顿第二定律
惯性力
运动的关联
微分方程的动力学应用
例如,带空气阻力的落体问题。
动力学方程[math]\displaystyle{ ma = mg - kv }[/math]
化为:[math]\displaystyle{ m\ddot{x} = mg - k\dot{x} }[/math]
[math]\displaystyle{ \int\frac{m\ddot{x}}{mg-k\dot{x}}\ dt = \int dt }[/math]
[math]\displaystyle{ \int\frac{dv}{mg-kv} = \int \frac{dt}{m} }[/math]
[math]\displaystyle{ -\frac{1}{k}\ln\left(mg-kv\right) = \frac{t}{m} + C_0 }[/math]
[math]\displaystyle{ mg - kv = e^{-\frac{k}{m}t + C_1} }[/math]
[math]\displaystyle{ v = \frac{mg}{k} + Ce^{-\frac{k}{m}t} }[/math]
且[math]\displaystyle{ v(0) = 0 }[/math]
所以[math]\displaystyle{ C = -\frac{mg}{k} }[/math]
所以求得[math]\displaystyle{ v = \frac{mg}{k}\left(1 - \exp\left\{-\frac{k}{m}t\right\}\right) }[/math]
能量
狭义功
保守力与非保守力
功能原理和机械能守恒定律
功能原理:系统的机械能等于系统内势能、动能和非保守力所做功以及系统所受外力做的功的总和,
即[math]\displaystyle{ E = E_k + E_p + A_{\textup{内非}} + A_{\textup{外}} }[/math]
机械能守恒定律:当系统不受外力,系统内不受非保守力时,该系统机械能守恒,
即[math]\displaystyle{ E_p + E_k = const }[/math]
或[math]\displaystyle{ \Delta E_p + \Delta E_k = 0 }[/math]
质心系与柯尼希定理
守恒量代替自由度
动量、角动量
有关的所有公式、定律、定理
动量:[math]\displaystyle{ p = mv }[/math]
冲力:[math]\displaystyle{ F = \lim_{\Delta t \to 0}{\frac{m \Delta v}{\Delta t}} = ma }[/math]
动量定理:[math]\displaystyle{ \sum{I_i} = \sum {m_i v_i} }[/math](这里[math]\displaystyle{ I }[/math]是冲量,量纲同动量)
动量守恒定律:系统在不受外力的情况下动量矢量和为零,即[math]\displaystyle{ \sum {m_i v_i} = 0 }[/math]
转动惯量[math]\displaystyle{ I }[/math](下面使用[math]\displaystyle{ I }[/math]的都是转动惯量)
常见物体的转动惯量,请见[[1]]
角加速度:[math]\displaystyle{ \beta = \lim_{\Delta t \to 0}{\frac{\Delta \omega}{\Delta t}} }[/math]
角动量:[math]\displaystyle{ L = r\times p = I\omega }[/math]
角动量守恒定律:在转动系统中,若物体只受有心力则角动量守恒,即[math]\displaystyle{ L = \textup{恒量} }[/math]
力矩:[math]\displaystyle{ M = \lim_{\Delta t \to 0}{\frac{\Delta L}{\Delta t}} = I\beta }[/math]
平行轴定理:设刚体质量为[math]\displaystyle{ m }[/math],选择过刚体质心的转动轴时刚体的转动惯量为[math]\displaystyle{ I_0 }[/math],则相对于距离该转轴为[math]\displaystyle{ d }[/math]的转轴,有[math]\displaystyle{ I = I_0 + md^2 }[/math]
刚体转动动能:[math]\displaystyle{ E_k = \frac{1}{2}I\omega^2 }[/math]
刚体平衡条件:平动[math]\displaystyle{ \sum{F_i} = 0 }[/math],转动:[math]\displaystyle{ \sum{M_i} = 0 }[/math]
守恒律
从古至今,物理中最美妙的就是守恒与不守恒。
目前,人们发现的大多是守恒律。
比如,能量守恒、动量守恒、角动量守恒、电荷守恒,以及霍金等人的猜想:信息守恒。
朗道曾经说:能量守恒体现时间平移对称性,动量守恒体现空间平移对称性,角动量守恒体现空间转动对称性,电荷守恒体现整体规范对称性。具体怎么证请参考《朗道物理学教程:力学》
但是,不守恒也有它的独到之处,宇称不守恒就诠释了宇宙在弱力作用下,正反物质不守恒,这才导致了物质湮灭时物质更占优势,而这万分之一的优势则构成了现在宇宙。
分析力学
定义广义动能[math]\displaystyle{ E_k }[/math],广义势能[math]\displaystyle{ E_p }[/math],广义位移[math]\displaystyle{ x }[/math],广义速度[math]\displaystyle{ v }[/math]
对于机械能守恒的系统,可以定义拉格朗日函数[math]\displaystyle{ L = E_k - E_p }[/math]
则运动体系满足[math]\displaystyle{ \frac{d}{dt}\frac{\partial L}{\partial v}=\frac{\partial L}{\partial x} }[/math]
分析力学适用于单个物体处于低自由度的复杂体系,整个系统内部物体可以使用偏微分方程联立。
例如,对于单摆,我们有广义动能[math]\displaystyle{ E_k = \frac{1}{2}mv^2=\frac{1}{2}ml^2\omega^2 }[/math],广义势能[math]\displaystyle{ E_p=mgl\left(1-\cos\theta\right) }[/math],广义位移[math]\displaystyle{ \theta }[/math],广义速度[math]\displaystyle{ \omega }[/math],广义加速度[math]\displaystyle{ \beta }[/math].
可得拉格朗日函数[math]\displaystyle{ L = E_k - E_p = \frac{1}{2}ml^2\omega^2 - mgl\left(1-\cos\theta\right) = \frac{1}{2}ml^2\omega^2 + mgl\cos\theta - mgl }[/math]
由[math]\displaystyle{ \frac{d}{dt}\frac{\partial L}{\partial \omega}=\frac{\partial L}{\partial \theta} }[/math]得:
[math]\displaystyle{ \frac{d}{dt}ml^2\omega=-mgl\sin\theta }[/math]
[math]\displaystyle{ ml\beta+mg\sin\theta=0 }[/math]
在微小摆动下,可以简化为[math]\displaystyle{ ml\beta+mg\theta=0 }[/math]
就是最常见的简谐振动的微分方程形式[math]\displaystyle{ ml\ddot{\theta}+mg\sin\theta=0 }[/math]
可以轻松得到单摆的一系列性质[math]\displaystyle{ T = 2\pi\sqrt{\frac{l}{g}}, \theta = \theta_{max}\cos{\sqrt{\frac{g}{l}}t} }[/math]等等。
天体运动
开普勒三定律
第一定律(轨道定律),行星的运动轨道是椭圆,太阳就位于椭圆的一个焦点上。
第二定律(面积定律):行星的运动在相同时间内扫过的面积是相等的[math]\displaystyle{ \vec{S_v} = \frac{1}{2} \vec{v} \times \vec{r} = const }[/math]。
第三定律(调和定律):周期的平方和椭圆半长轴的立方的比值是定值[math]\displaystyle{ \frac{a^3}{T^2} = k }[/math]
第一定律中,椭圆轨道与万有引力是平方反比力息息相关。
第二定律中,[math]\displaystyle{ \vec{S_v} = \frac{1}{2}\vec{v}\times\vec{r} = \frac{L}{2m} = const }[/math],其本质是角动量守恒。
第三定律可以由第一第二定律直接推导出来。
[math]\displaystyle{ T = \frac{S}{S_v} = \frac{\pi ab}{\frac{1}{2}vr\sin\theta} = \frac{2\pi ab}{vr\sin\theta} = \frac{2\pi mab}{L} }[/math]
[math]\displaystyle{ \frac{1}{2}mv_1^2 - \frac{GMm}{r_1} = \frac{1}{2}mv_2^2 - \frac{GMm}{r_2} }[/math]
[math]\displaystyle{ r_1 + r_2 = 2a, r_1r_2 = b^2 }[/math]
可以解得:[math]\displaystyle{ S_v = \frac{b}{2}\sqrt{\frac{GM}{a}} }[/math]
[math]\displaystyle{ T = \frac{S}{S_v} = \frac{\pi ab}{\frac{b}{2}\sqrt{\frac{GM}{a}}} = 2\pi a\sqrt{\frac{a}{GM}} }[/math]
所以[math]\displaystyle{ \frac{a^3}{T^2} = \frac{GM}{4\pi^2} = k }[/math]
平方反比引力
一个力之所以是平方反比,是因为它激发的场的能量在三维空间中有各向同性的性质,所以它的能量衰减与[math]\displaystyle{ 4\pi r^2 }[/math]成反比。
之前说由平方反比力引决定了行星轨道是椭圆。现在反过来,由椭圆轨道推导平方反比引力。
椭圆方程[math]\displaystyle{ r = \frac{P}{1+e\cos\theta} }[/math]
改写成[math]\displaystyle{ \frac{1}{r} = \frac{1}{P} + \frac{e}{P}\cos\theta }[/math]
令[math]\displaystyle{ \mu = \frac{1}{r},h=r^2\theta }[/math]
运用比耐公式[math]\displaystyle{ h^2\mu^2\left(\frac{d^2\mu}{d\theta^2}+\mu\right)+\frac{F}{m} = 0 }[/math]
所以[math]\displaystyle{ F = -mh^2\mu^2\left(\frac{d^2\mu}{d\theta^2}+\mu\right)=-\frac{h^2}{P}\frac{m}{r^2} }[/math]
这就表明了行星所受的是平方反引力。
万有引力定律
接上一小节,在证明了万有引力是平方反比引力后,我们进一步推导。
由开普勒第三定律:[math]\displaystyle{ \frac{a^3}{T^2} = \frac{h^2}{4\pi^2P} }[/math]
虽然[math]\displaystyle{ h,P }[/math]是和行星有关的常数,但是根据开普勒第三定律[math]\displaystyle{ \frac{h^2}{P} }[/math]是一个与行星无关的常数。
于是,令[math]\displaystyle{ \frac{h^2}{P}=k^2 }[/math],就可以把[math]\displaystyle{ F = -\frac{h^2}{P}\frac{m}{r^2} }[/math]化为[math]\displaystyle{ F = -\frac{k^2m}{r^2} }[/math]
但是力的作用是相互的,因此万有引力不仅与行星质量成正比,还应与中心天体质量成正比,于是[math]\displaystyle{ F\propto -\frac{Mm}{r^2} }[/math]
令:[math]\displaystyle{ G = \frac{k^2}{M} }[/math],就可以得到[math]\displaystyle{ F = -\frac{GMm}{r^2} }[/math]
也可以写成矢量式[math]\displaystyle{ \vec{F} = -\frac{GMm}{r^2}\hat{r} = -\frac{GMm}{r^2}\frac{\vec{r}}{r} }[/math]
三个宇宙速度
振动与波动
线性常微分方程
振动
定义
简谐振动
受迫振动
阻尼振动
简谐振动的判定方法
振动的合成与分解
波动
横波
纵波
波的合成与分解
马赫锥
切伦科夫辐射
多普勒效应
电磁学
静电学
电荷及其守恒定律
两种电荷
静电感应
电荷守恒定律
在任何物理过程中,电荷的代数和是守恒的,这叫做电荷守恒定律。
库仑定律
万有引力与静电力
万有引力,服从半径平方衰减的规律。
静电力也是吗?(当然)
除此之外,甚至还有更有意思的现象,比如薄球壳内的物体不受万有引力,带电的薄球壳中的带电体也不受静电力。
在静电力中,我们称之为静电屏蔽(《三体》里面人类就是这么屏蔽三体人的质子的)
我们可以在这里证明万有引力的“球壳屏蔽”现象:

设均匀薄球壳质量的面密度为[math]\displaystyle{ \sigma }[/math],设在球壳内任意一点[math]\displaystyle{ A }[/math]处有一个质量为[math]\displaystyle{ m }[/math]的质点。在球壳上去一个很小的面元[math]\displaystyle{ \Delta S_1 }[/math],它的质量[math]\displaystyle{ \Delta m_1 = \sigma\Delta S_1 }[/math],它与[math]\displaystyle{ A }[/math]点距离为[math]\displaystyle{ r_1 }[/math],则此面元对于[math]\displaystyle{ A }[/math]处的万有引力为:
[math]\displaystyle{ \Delta F_1 = \frac{G m\Delta m_1}{r_1^2} = \frac{G\sigma m\Delta S_1}{r_1^2} }[/math]
反向延长这两条线,可以得到另一个面元[math]\displaystyle{ \Delta S_2 }[/math],于是我们可以将面元[math]\displaystyle{ \Delta S_1、\Delta S_2 }[/math]看成线段。
故在这个圆面中,由相交弦定理可得[math]\displaystyle{ A-\Delta S_1 }[/math]与[math]\displaystyle{ A-\Delta S_2 }[/math]两个三角形相似。
故有:[math]\displaystyle{ \frac{\Delta S_1}{\Delta S_2} = \frac{r_1^2}{r_2^2} }[/math]
且对于面元[math]\displaystyle{ \Delta S_2 }[/math]在[math]\displaystyle{ A }[/math]处的万有引力为:
[math]\displaystyle{ \Delta F_2 = \frac{G m\Delta m_2}{r_2^2} = \frac{G\sigma m\Delta S_2}{r_2^2} }[/math]
由此可知:[math]\displaystyle{ \Delta F_1 = \Delta F_2 }[/math]
且由于两处面元所提供的的万有引力正好方向相反,所以其合力为零,同理,在各个方向上的万有引力合力都是零,故质点[math]\displaystyle{ m }[/math]所受万有引力力为零。
库仑定律
设两个电荷的电量为[math]\displaystyle{ q_1,q_2 }[/math],距离为[math]\displaystyle{ r }[/math],则库仑力大小为:[math]\displaystyle{ F = k_e \frac{q_1 q_2}{r^2} }[/math]
其中:[math]\displaystyle{ k_e }[/math]是静电力常量,在真空中常常写成[math]\displaystyle{ k_e = \frac{1}{4\pi\varepsilon_0} }[/math],[math]\displaystyle{ \varepsilon_0 }[/math]被称为真空介电常量。
通常,[math]\displaystyle{ k_e = 9.0\times 10^9 \textup{N}\cdot \textup{m}^2/\textup{C}^2 }[/math],[math]\displaystyle{ \varepsilon_0 = 8.85 \times 10^{-12} \textup{C}^2/\textup{N}\cdot \textup{m}^2 }[/math]。
当然,文字表述为:在真空中,两个静止的点电荷[math]\displaystyle{ q_1、q_2 }[/math]之间的相互作用力的大小和[math]\displaystyle{ q_1、q_2 }[/math]的乘积成正比,和它们之间的距离[math]\displaystyle{ r }[/math]的平方成反比;作用力方向沿着它们的联线,同号电荷相斥,异号电荷相吸。
当然,和万有引力一样,库仑力服从力的叠加原则。
各种基本作用力的进一步的对比
电场 电势
梯度
散度
旋度
电通量
高斯定理
电偶极子
等势面
电像法
带电粒子在电场中的运动
静电场中的导体
电容、电容器
电介质
极化
电极化强度矢
电位移矢量
电路
大家都知道,静电场的缺点就是无法维持一个恒定的电势。在静电平衡后,就再也没有电流流动了,所以我们需要一个恒定的电势来维持一个稳定的电流。
那么什么东西可以做到呢?那就是直流电源,我们叫作电池。
电流
电流的本质
电流密度
电阻
电导率 电阻率
等效电阻
电势
电动势
等效电压源
等效电流源
等效电动势
欧姆定律
基尔霍夫定律
稳恒电路
带容电路
静磁
磁的本质
磁感应强度
磁感应强度
磁场与电场的镜面对称
磁通量
磁高斯定理
毕奥萨伐尔定律
对于一个闭合回路的电流圈,在空间中一点处的磁感应强度可以写为:
[math]\displaystyle{ \vec{B} = \oint_L\frac{\mu_0}{4\pi}\frac{Id\vec{l}}{r^2}\times\hat{r} }[/math]
安培环路定理
环路积分
洛伦兹力
安培力
磁矩
磁偶极子
行列式 基矢
带电粒子的运动 力电磁综合
磁聚焦 磁透镜
常微分方程组
磁场中的守恒量
轨道拼接
电磁感应
麦克斯韦方程组
磁生电
涡旋电场
感生电动势
动生电动势
电感
自感
互感
感抗 容抗
电学自由度
电磁动力学
交变电路
交流电源
复变交流电 复电路
光学
几何光学
波动光学
热学
热学基本概念
物质的聚集态
热力学定律
指正化学高考错题
我们知道,二氧化氮与四氧化二氮在压强不同的情况下可以相互转化,产生一组热力学的动态平衡。
二氧化氮是红棕色气体,而四氧化二氮无色。
现在将混合气体放在一密闭注射器中,向里推活塞,请问气体颜色变深还是变浅?
近代物理学
近代物理学是人们打破常识,将物理学科向更加纵深发展的成果。
其中发展主要聚集在20世纪前半叶,这也是科学发展的黄金时代。
知识补充
首先,狭义相对论是电磁学研究与经典力学研究发生严重冲突时引起的对物理体系的一次大修正。
所以,首先要知道著名的麦克斯韦方程组。
第一个式子为静电高斯定律,第二个为静磁高斯定律,第三个为法拉第电磁感应定律,第四个为安培—麦克斯韦定律。
微分形式:
[math]\displaystyle{ \nabla\cdot\vec{E} = \frac{\rho}{\varepsilon_0} }[/math]
[math]\displaystyle{ \nabla\cdot\vec{B} = 0 }[/math]
[math]\displaystyle{ \nabla\times\vec{E} = -\frac{\partial\vec{B}}{\partial t} }[/math]
[math]\displaystyle{ \nabla\times\vec{B} = \mu_0 \vec{j} + \frac{\partial\vec{E}}{\partial t} }[/math]
积分形式:
[math]\displaystyle{ \oiint{\vec{E}\cdot\textup{d}\vec{s}} = \frac{Q}{\varepsilon_0} }[/math]
[math]\displaystyle{ \oiint{\vec{B}\cdot\textup{d}\vec{s}} = 0 }[/math]
[math]\displaystyle{ \oint_{L}{\vec{E}\cdot\textup{d}\vec{l}} = -\frac{\textup{d}\Phi_B}{\textup{d}t} }[/math]
[math]\displaystyle{ \oint_{L}{\vec{B}\cdot\textup{d}\vec{l}} = \mu_0 I + \frac{\textup{d}\Phi_E}{\textup{d}t} }[/math]
狭义相对论
历史因缘
到了19世纪,物理学家法拉第凭借自己惊人的物理直觉极大的推动了电磁学的发展。
但是,他数学不好。
因此,当他隐隐感觉到有“电磁波”这样的东西时,他却无法得到电磁波的精确解 (哈!让你不学数学!)
于是,他找到了麦克斯韦,这位伟大的数学家。
在法拉第的研究基础上,麦克斯韦推出了麦克斯韦方程组,从数学上预言了电磁波的存在。
结果,问题来了。
麦克斯韦方程组指出电磁波的速度是光速。
而且,经典物理学的发展过程中,人们有两大理论支柱支撑:
第一,在任何惯性参考系下,所有物理定律的数学形式都是一样的(牛顿第二定律就是牛顿第二定律,运动方程就是运动方程,换了参考系也不会变的)
第二,是伽利略相对性原理,就是关于相对速度的计算问题(就是小学的行程问题中的相对速度)
我们是否可以认为,当我们跑得和光一样快,我们可以看到停滞的电磁波?
可是麦克斯韦方程组在大量实验中也通过了考验,证明了其正确性,再由第一条,我们可知道电磁波在任何参考系下的速度都是光速。
而这和第二条违背。
当时著名的迈克尔逊-莫雷实验测出了在地球绕太阳运转时法向和切向相对于光的速度是一样的,更加让人们百思不得其解。 (地球绕太阳运转的切向速度是[math]\displaystyle{ 2.98\times 10^4 \textup{m}/\textup{s} }[/math],而法向速度可以看做是零,可是这样大的差别竟然没有任何一个精密仪器可以测出来!)
那么到这里,答案呼之欲出。
爱因斯坦的思考路径
爱因斯坦也想到这里,列出了上述的三条结论。
他认为:既然矛盾,那就必然有一个错了。
首先,他思考了第一个结论,如果第一个结论是错的,那么他就要找出一个不同于其他惯性系的惯性系,可是惯性系有无穷多个,又怎么能找到一个特殊的惯性系呢?况且找到了,也会违反物理的普适性。
接着,他在确立第一个结论是不会错的基础上,知道麦克斯韦方程组和伽利略相对性原理存在矛盾,它们之中必然有一个错了。
那么哪个错了呢?
爱因斯坦当然是选择了麦克斯韦方程组(毕竟搞点反常识的东西,才是科学发展的动力)
于是,他提出了狭义相对论的两条进本原则(或者说基本假设)
- 所有的参考系都是平权的
- 在所有的参考系中,电磁波的速度(光速)保持不变,叫做光速不变性。
基于两条基本原则的数学推导
钟慢效应
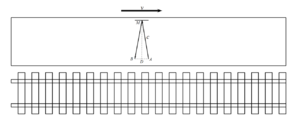
如图所示,我们设想有一列火车以速度[math]\displaystyle{ v }[/math]向右行驶,这时从[math]\displaystyle{ A }[/math]点发出一道激光,假设[math]\displaystyle{ M }[/math]处有一面镜子,光在[math]\displaystyle{ M }[/math]处被反射至[math]\displaystyle{ B }[/math]点,假设大地上的人这一过程花费了时间[math]\displaystyle{ \Delta t }[/math],车上的人认为时间过了[math]\displaystyle{ \Delta t' }[/math],光速为[math]\displaystyle{ c }[/math]。
我们知道,在车厢中的人看到这束激光必定是原路返回的,即[math]\displaystyle{ A、B }[/math]两点是重合的,而在大地系上的人来看(或者相对于铁轨),光却走过了一个锯齿状的路线。
设这段路线的长度为[math]\displaystyle{ 2l }[/math],且我们知道[math]\displaystyle{ MA = MB = l = \sqrt{MD^2 + \left(\frac{v\Delta t}{2}\right)^2} }[/math],则[math]\displaystyle{ MA + MB = 2l = \sqrt{4MD^2 + \left(v\Delta t\right)^2} = c\Delta t }[/math]
而在车上的人认为这道激光走了[math]\displaystyle{ 2MD = c\Delta t' }[/math]。
消去[math]\displaystyle{ MD }[/math]之后我们可得出这样一个式子:[math]\displaystyle{ \Delta t' = \frac{\Delta t}{\sqrt{1-\frac{v^2}{c^2}}} }[/math]。
我们发现,车上的人相对于大地系中的人来讲,时间变慢了,这就称为钟慢效应。
尺缩效应
我们首先测量静止时的长度,设测得的长度为[math]\displaystyle{ L_0 = \frac{c\Delta t}{2} }[/math]。
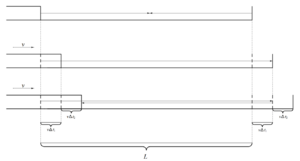
下面,我们把推导分成两个阶段。
第一阶段,左侧的激光发射器发出一道激光,整个装置向右移动速度为[math]\displaystyle{ v }[/math]。
那么我们可以得到激光出程开始直到抵达镜面的时间[math]\displaystyle{ \Delta t_1 = \frac{L}{c - v} }[/math]。
在此期间,整个装置向前移动了[math]\displaystyle{ v\Delta t_1 = \frac{Lv}{c-v} }[/math],
第二阶段,激光被反射,回到正在前进的装置左端,接收的时间花费了时间[math]\displaystyle{ \Delta t_2 = \frac{L}{c + v} }[/math]
两段总时间为[math]\displaystyle{ \Delta t' = \Delta t_1 + \Delta t_2 = \frac{2cL}{c^2 - v^2} = \frac{2L}{c\left(1 - \frac{v^2}{c^2}\right)} }[/math]。
结合可知[math]\displaystyle{ \frac{\Delta t'}{\Delta t} = \frac{L}{L_0\left(1 - \frac{v^2}{c^2}\right)} = \frac{1}{\sqrt{1-\frac{v^2}{c^2}}} }[/math]。
这样我们解得[math]\displaystyle{ L = L_0\sqrt{1-\frac{v^2}{c^2}} }[/math]。
这说明,在大地系的人的眼中,这个运动的装置长度缩短了。
闵氏时空
闵氏时空是爱因斯坦的数学老师闵可夫斯基创造的时空坐标系,从数学的角度极大的简化了狭义相对论的物理过程。
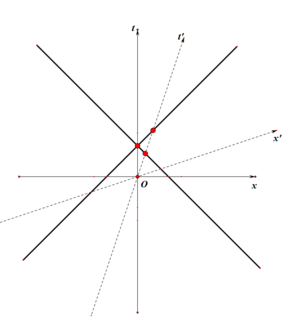
首先,闵可夫斯基建立了一个平面之间坐标系,横轴是空间轴,又叫[math]\displaystyle{ x }[/math]轴,纵轴是时间轴,又叫[math]\displaystyle{ ct }[/math]轴,通常只写作[math]\displaystyle{ t }[/math],之所以在时间上乘以一个光速,为的是让以光速运动的物体在坐标轴中的轨迹始终是象限的角平分线(或者说,在狭义相对论中,我们规定光速[math]\displaystyle{ c = 1 }[/math],这样可以简化很多问题的计算)。
由于狭义相对论只是对速度的问题作了讨论,因此不考虑加速问题,所以闵氏时空和狭义相对论的时空必须一致,即都是平直的,也都只能研究匀速运动的问题。
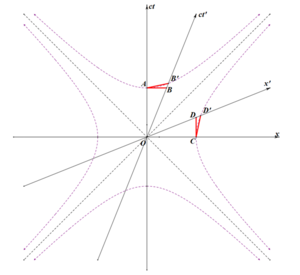
在一个参考系相对于另一个参考系匀速运动时,它会发生收缩,即洛伦兹变换,那么对应着闵氏时空,这个参考系的坐标系也会相应的收缩,就像图中所示的坐标系[math]\displaystyle{ x'Oct' }[/math]。
这里运动参考系的坐标系收缩时,时间轴在静止系中的斜率为[math]\displaystyle{ ±\frac{c}{v} }[/math],空间轴在静止系中的斜率为[math]\displaystyle{ ±\frac{v}{c} }[/math],它们同正同负,具体正负应依照相对运动的方向来定。
我们把一个物体上发生物理过程用一条曲线或直线来表示,这条线被称作这个物体的世界线。
最值得一提的是,在闵氏时空中,规定:两点之间直线最长,因为闵氏时空的距离公式为[math]\displaystyle{ d = \sqrt{\left|\left(t_1-t_2\right)^2 - \left(x_1-x_2\right)^2\right|} }[/math],一般情况下,我们把一个事件的开始点设在原点处,所以距离公式一般简写作[math]\displaystyle{ d = \sqrt{\left|t^2-x^2\right|} }[/math]
所以不难发现,等长线段必须要通过双曲线才能在图上直观比较出距离的大小。
物理上把这四支双曲线称之为闵氏时空的校准曲线。
因为闵氏时空下的距离公式中根号下带绝对值,所以不难推得,当[math]\displaystyle{ d = \sqrt{t^2-x^2} }[/math]时,这个物理过程更靠近时间轴,所以对于这种情况,称之为类时的;而当[math]\displaystyle{ d = \sqrt{x^2-t^2} }[/math]时,称这个物理过程为类空的。
类时时空由于比光的世界线更靠近时间轴,所以在原点一个物体可以用一段连续曲线所表示的物理过程来到达位于类时时空中的点(可以称之为一个事件),即速度小于光速;反之,类空时空中的速度由于超越了光速,所以这个物体是没有办法自己到达这个点的,不过随着时间的推移,类空时空会因为空间轴在时间上平移而变为类时时空,但这个物体所观测到这个事件时,这个事件已经发生过一段时间了。
光的世界线就是类时和类空的临界,因为类时时空很像沙漏,所以类时时空就是大名鼎鼎的“时光锥”,在锥内的事件是人们可控的,锥外的则不是。
显然,发明这个时空坐标系是有很大用处的,我们看图:根据校准曲线,我们可以看到[math]\displaystyle{ OA }[/math]比[math]\displaystyle{ OB }[/math]更长,而同作为描述时间长度的线段,我们便可以知道,运动的参考系比静止的参考系时间走得更慢一些,这就是钟慢效应的几何解释。同理,我们可以看到尺缩效应在这张图上被完美诠释了。
关于狭义相对论的几个基本问题
对钟
对钟是指在同一个惯性系内,所有的标准钟都可以对到同一示数。
我们设想有两个人相距非常遥远,两个手中各有一只钟,其中一个人在[math]\displaystyle{ a }[/math]时刻发出一道激光,而另一个人用镜子将其反射,原路返回,直到第一个人在[math]\displaystyle{ b }[/math]时刻接受到信号,于是他可以推算出对方的接收到该信号的时刻为[math]\displaystyle{ \frac{a + b}{2} }[/math],此时他打一个电话告诉另外一个人自己的所作所为,那个人就可以根据经过的时间,把钟的时刻对的和他一模一样。
对钟问题是解决下面三个问题的基础。
闪电击中火车问题/同时的相对性
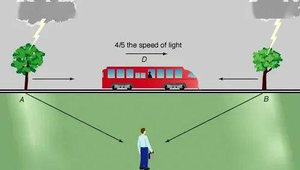
有一辆火车向右以速度[math]\displaystyle{ v }[/math]行驶,此时,大地系中,火车头尾同时各劈下一道闪电,此时,火车中点处站了一个人,与他同一位置的大地上也站了一个人,两人在此时已经将钟对好。
如果,以大地系中的人来看,由于他距离两闪电劈下来的位置的距离相等,因此他是同时接收到这两个闪电的。
那么,在火车上的人呢?
首先,如果以大地系来看,那么这个人显然会先收到来自车头的光,然后隔一段时间后再接收到车尾的光。
如果以车系来看呢?
首先,要让大地系中的人知道自己是否接受到了其中的一束光。
我们可以假设大地上在每一个位置上都站了一个人,这些人都已经对好了钟,火车中的人在接收到光时,瞬时向大地系中的人报告这一事件。因此,大地系中的人可以准确的知道车中的人先接收到车头的光,再收到车尾的光。
那么事实就是,车中的人确实没有同时收到两束光。
但是以车为系时,车中的人看这两束光向自己飞来的速度都是一样的,这是相对论的基本假设。
且由于此人站在火车中点,所以两束光到此处的距离一样,因此每一束光到达此人所用的时间也应该一样。
而闪电是同时劈到车头车尾的,所以按理说,两束光到达人的时间应该是一样的。 出现矛盾。
由于相对论的假设是得到了大量证实的,所以第一条,每一束光到人所用的时间确实一样,所以为了不矛盾,我们就能得出一个看似匪夷所思的结论:在车系中,这两束闪电并非同时劈下来。
在同一个惯性系发生的同时事件,在另一个惯性系中就可能不是同时的,这就叫做同时的相对性。
也可以用闵氏时空图来判断。
在图中,我们清楚地观察到在车头位置的光的世界线与车系的时间轴相交的位置代表的时间点更早。
如果画两条校准曲线,也可以看到,在大地系中的人也是认为车上的人更先接收到车头的光。
双生子佯谬
车库佯谬
量子力学
原子物理
| 数学 |
| ||||
|---|---|---|---|---|---|
| 物理 |
| ||||
| 化学 |
| ||||
| 信息学 |
| ||||
| 生物学 |
| ||||
| 其他 | |||||
